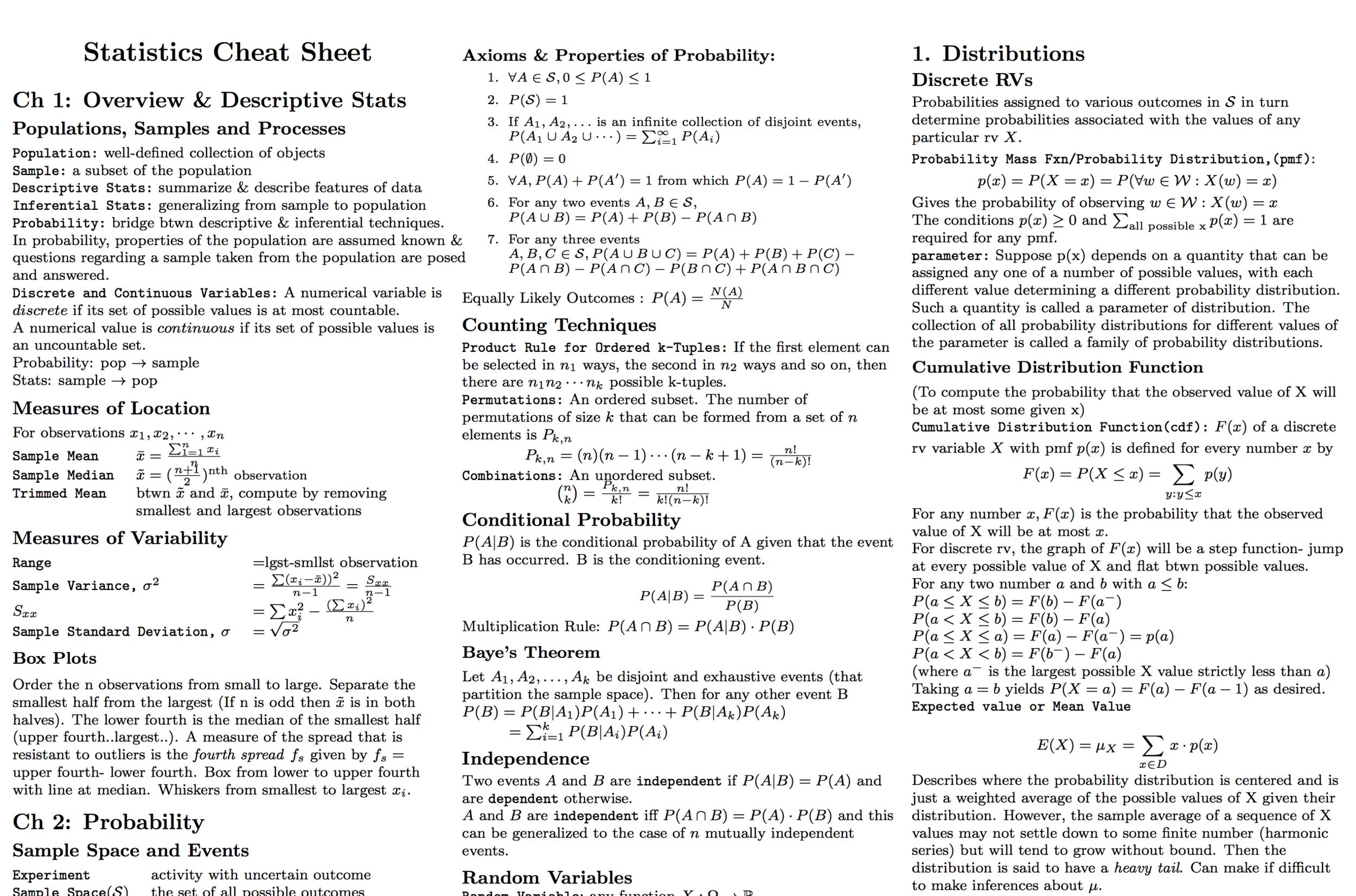
Then, download my handy statistics cheat sheet! My statistics cheat sheet has every statistical function and formula you will need to calculate uncertainty and evaluate your results. All packed into 2 pages! Check out all of the great functions that I packed into this statistics cheat sheet. Average Variance Standard Deviation Determining. Statistics Cheat Sheet Population The entire group one desires information about Sample A subset of the population taken because the entire population is usually too. Calculation of Statistics - STAT CALC. 1. 1-Var Stats - used to find important statistical information with 1 variable ¥ assumes that the list that the data you want information about is in L1. If not, you must specify it (ex. 1-Var Stats L3) ¥ The following information is given: x - the numerical mean (average)! X - the sum of your data 2. Important Concepts not on the AP Statistics Formula Sheet Part I: IQR = Q 3 – Q 1 Test for an outlier: 1.5(IQR) above Q 3 or below Q 1 The calculator will run the test for you as long as you choose the boxplot with the oulier on it in STATPLOT Linear transformation: Addition: affects center NOT spread adds to, M, Q 1, Q 3, IQR not σ. Probability Cheat Sheet Distributions Unifrom Distribution notation Ua;b cdf x a b a for x2a;b pdf 1 b a for x2a;b expectation 1 2 (a+ b) variance 1 12 (b a)2 mgf etb eta t(b a) story: all intervals of the same length on the distribution’s support are equally probable. Gamma Distribution notation Gamma(k; ) pdf kx 1e x ( k) I x0 ( k.
By Afshine Amidi and Shervine Amidi
Parameter estimation
Definitions
Random sample A random sample is a collection of $n$ random variables $X_1, ..., X_n$ that are independent and identically distributed with $X$.
Estimator An estimator is a function of the data that is used to infer the value of an unknown parameter in a statistical model.
Bias The bias of an estimator $hat{theta}$ is defined as being the difference between the expected value of the distribution of $hat{theta}$ and the true value, i.e.:
Remark: an estimator is said to be unbiased when we have $E[hat{theta}]=theta$.
Estimating the mean
Sample mean The sample mean of a random sample is used to estimate the true mean $mu$ of a distribution, is often noted $overline{X}$ and is defined as follows:
Remark: the sample mean is unbiased, i.e $E[overline{X}]=mu$.
Characteristic function for sample mean The characteristic function for a sample mean is noted $psi_{overline{X}}$ and is such that:
Central Limit Theorem Let us have a random sample $X_1, ..., X_n$ following a given distribution with mean $mu$ and variance $sigma^2$, then we have:
Estimating the variance
Sample variance The sample variance of a random sample is used to estimate the true variance $sigma^2$ of a distribution, is often noted $s^2$ or $hat{sigma}^2$ and is defined as follows:
Remark: the sample variance is unbiased, i.e $E[s^2]=sigma^2$.
Chi-Squared relation with sample variance Let $s^2$ be the sample variance of a random sample. We have:
Confidence intervals
Definitions
Confidence level A confidence interval with confidence level $1-alpha$ is such that $1-alpha$ of the time, the true value is contained in the confidence interval.
Confidence interval A confidence interval $CI_{1-alpha}$ with confidence level $1-alpha$ of a true parameter $theta$ is such that:
With the notation of the example above, a possible $1-alpha$ confidence interval for $theta$ is given by $CI_{1-alpha} = [x_1, x_2]$.
Stats Cheat Sheets And Formulas
Confidence interval for the mean
When determining a confidence interval for the mean $mu$, different test statistics have to be computed depending on which case we are in. The table below sums it up.
| Distribution of $X_i$ | Sample size $n$ | Variance $sigma^2$ | Statistic | $small 1-alpha$ confidence interval |
| $X_isimmathcal{N}(mu, sigma)$ | any | known | $displaystylefrac{overline{X}-mu}{frac{sigma}{sqrt{n}}}simmathcal{N}(0,1)$ | $left[overline{X}-z_{frac{alpha}{2}}frac{sigma}{sqrt{n}},overline{X}+z_{frac{alpha}{2}}frac{sigma}{sqrt{n}}right]$ |
| $X_isim$ any distribution | large | known | $displaystylefrac{overline{X}-mu}{frac{sigma}{sqrt{n}}}simmathcal{N}(0,1)$ | $left[overline{X}-z_{frac{alpha}{2}}frac{sigma}{sqrt{n}},overline{X}+z_{frac{alpha}{2}}frac{sigma}{sqrt{n}}right]$ |
| $X_isim$ any distribution | large | unknown | $displaystylefrac{overline{X}-mu}{frac{s}{sqrt{n}}}simmathcal{N}(0,1)$ | $left[overline{X}-z_{frac{alpha}{2}}frac{s}{sqrt{n}},overline{X}+z_{frac{alpha}{2}}frac{s}{sqrt{n}}right]$ |
| $X_isimmathcal{N}(mu, sigma)$ | small | unknown | $displaystylefrac{overline{X}-mu}{frac{s}{sqrt{n}}}sim t_{n-1}$ | $left[overline{X}-t_{frac{alpha}{2}}frac{s}{sqrt{n}},overline{X}+t_{frac{alpha}{2}}frac{s}{sqrt{n}}right]$ |
| $X_isim$ any distribution | small | known or unknown | Go home! | Go home! |
Note: a step by step guide to estimate the mean, in the case when the variance in known, is detailed here.
Confidence interval for the variance
The single-line table below sums up the test statistic to compute when determining the confidence interval for the variance.
| Distribution of $X_i$ | Sample size $n$ | Mean $mu$ | Statistic | $small 1-alpha$ confidence interval |
| $X_isimmathcal{N}(mu,sigma)$ | any | known or unknown | $displaystylefrac{s^2(n-1)}{sigma^2}simchi_{n-1}^2$ | $left[frac{s^2(n-1)}{chi_2^2},frac{s^2(n-1)}{chi_1^2}right]$ |
Note: a step by step guide to estimate the variance is detailed here.
Hypothesis testing
General definitions
Type I error In a hypothesis test, the type I error, often noted $alpha$ and also called 'false alarm' or significance level, is the probability of rejecting the null hypothesis while the null hypothesis is true. If we note $T$ the test statistic and $R$ the rejection region, then we have:
Type II error In a hypothesis test, the type II error, often noted $beta$ and also called 'missed alarm', is the probability of not rejecting the null hypothesis while the null hypothesis is not true. If we note $T$ the test statistic and $R$ the rejection region, then we have:
p-value In a hypothesis test, the $p$-value is the probability under the null hypothesis of having a test statistic $T$ at least as extreme as the one that we observed $T_0$. We have:
Remark: the example below illustrates the case of a right-sided $p$-value.
Non-parametric test A non-parametric test is a test where we do not have any underlying assumption regarding the distribution of the sample.
Testing for the difference in two means
The table below sums up the test statistic to compute when performing a hypothesis test where the null hypothesis is:
| Distribution of $X_i, Y_i$ | Sample size $n_X, n_Y$ | Variance $sigma_X^2, sigma_Y^2$ | Test statistic under $H_0$ |
| Normal | any | known | $displaystylefrac{(overline{X}-overline{Y})-delta}{sqrt{frac{sigma_X^2}{n_X}+frac{sigma_Y^2}{n_Y}}}underset{H_0}{sim}mathcal{N}(0,1)$ |
| Normal | large | unknown | $displaystylefrac{(overline{X}-overline{Y})-delta}{sqrt{frac{s_X^2}{n_X}+frac{s_Y^2}{n_Y}}}underset{H_0}{sim}mathcal{N}(0,1)$ |
| Normal | small | unknown with $sigma_X=sigma_Y$ | $displaystylefrac{(overline{X}-overline{Y})-delta}{ssqrt{frac{1}{n_X}+frac{1}{n_Y}}}underset{H_0}{sim}t_{n_X+n_Y-2}$ |
Testing for the mean of a paired sample

We suppose here that $X_i$ and $Y_i$ are pairwise dependent. By noting $D_i=X_i-Y_i$, the one-line table below sums up the test statistic to compute when performing a hypothesis test where the null hypothesis is:
| Distribution of $X_i, Y_i$ | Sample size $n=n_X=n_Y$ | Variance $sigma_X^2, sigma_Y^2$ | Test statistic under $H_0$ |
| Normal, paired | any | unknown | $displaystylefrac{overline{D}-delta}{frac{s_D}{sqrt{n}}}underset{H_0}{sim}t_{n-1}$ |
Testing for the median
Median of a distribution We define the median $m$ of a distribution as follows:
Sign test The sign test is a non-parametric test used to determine whether the median of a sample is equal to the hypothesized median.
By noting $Vunderset{H_0}{sim}mathcal{B}(n,p=frac{1}{2})$ the number of samples falling to the right of the hypothesized median, we have:
$―$ If $npgeqslant5$, we use the following test statistic:
$―$ If $np < 5$, we use the following fact:
$chi^2$ test
Goodness of fit test Let us have $k$ bins where in each of them, we observe $Y_i$ number of samples. Our null hypothesis is that $Y_i$ follows a binomial distribution with probability of success being $p_i$ for each bin.
We want to test whether modelling the problem as described above is reasonable given the data that we have. In order to do this, we perform a hypothesis test:
$chi^2$ statistic for goodness of fit In order to perform the goodness of fit test, we need to compute a test statistic that we can compare to a reference distribution. By noting $k$ the number of bins, $n$ the total number of samples, if we have $np_igeqslant5$, the test statistic $T$ defined below will enable us to perform the hypothesis test:
Trends test
Number of transpositions In a given sequence, we define the number of transpositions, noted $T$, as the number of times that a larger number precedes a smaller one.
Example: the sequence ${1,5,4,3}$ has $T=3$ transpositions because $5>4, 5>3$ and $4>3$
Test for arbitrary trends Given a sequence, the test for arbitrary trends is a non-parametric test, whose aim is to determine whether the data suggest the presence of an increasing trend:
If we note $x$ the number of transpositions in the sequence, the $p$-value is computed as:
Remark: the test for a decreasing trend of a given sequence is equivalent to a test for an increasing trend of the inversed sequence.
Statistics Cheat Sheet College
Regression analysis
In the following section, we will note $(x_1, Y_1), ..., (x_n, Y_n)$ a collection of $n$ data points.
Simple linear model Let $X$ be a deterministic variable and $Y$ a dependent random variable. In the context of a simple linear model, we assume that $Y$ is linked to $X$ via the regression coefficients $alpha, beta$ and a random variable $esimmathcal{N}(0,sigma)$, where $e$ is referred as the error. We have:
Regression estimation When estimating the regression coefficients $alpha, beta$ by $A, B$, we obtain predicted values $hat{Y}_i$ as follows:
Sum of squared errors By keeping the same notations, we define the sum of squared errors, also known as SSE, as follows:
Method of least-squares The least-squares method is used to find estimates $A,B$ of the regression coefficients $alpha,beta$ by minimizing the SSE. In other words, we have:
Notations Given $n$ data points $(x_i, Y_i)$, we define $S_{XY},S_{XX}$ and $S_{YY}$ as follows:
Least-squares estimates When estimating the coefficients $alpha, beta$ with the least-squares method, we obtain the estimates $A, B$ defined as follows:
Sum of squared errors revisited The sum of squared errors defined above can also be written in terms of $S_{YY}$, $S_{XY}$ and $B$ as follows:
Key results
When $sigma$ is unknown, this parameter is estimated by the unbiased estimator $s^2$ defined as follows:
The estimator $s^2$ has the following property:
The table below sums up the properties surronding the least-squares estimates $A, B$ when $sigma$ is known or not:
| Coefficient | Estimate | $sigma$ | Statistic | $1-alpha$ confidence interval |
| $alpha$ | $A$ | known | $frac{A-alpha}{sigmasqrt{frac{1}{n}+frac{overline{X}^2}{S_{XX}}}}simmathcal{N}(0,1)$ | $left[A-z_{frac{alpha}{2}}sigmasqrt{frac{1}{n}+frac{overline{X}^2}{S_{XX}}},A+z_{frac{alpha}{2}}sigmasqrt{frac{1}{n}+frac{overline{X}^2}{S_{XX}}}right]$ |
| $beta$ | $B$ | known | $frac{B-beta}{frac{sigma}{sqrt{S_{XX}}}}simmathcal{N}(0,1)$ | $left[B-z_{frac{alpha}{2}}frac{sigma}{sqrt{S_{XX}}},B+z_{frac{alpha}{2}}frac{sigma}{sqrt{S_{XX}}}right]$ |
| $alpha$ | $A$ | unknown | $frac{A-alpha}{ssqrt{frac{1}{n}+frac{overline{X}^2}{S_{XX}}}}sim t_{n-2}$ | $left[A-t_{frac{alpha}{2}}ssqrt{frac{1}{n}+frac{overline{X}^2}{S_{XX}}},A+t_{frac{alpha}{2}}ssqrt{frac{1}{n}+frac{overline{X}^2}{S_{XX}}}right]$ |
| $beta$ | $B$ | unknown | $frac{B-beta}{frac{s}{sqrt{S_{XX}}}}sim t_{n-2}$ | $left[B-t_{frac{alpha}{2}}frac{s}{sqrt{S_{XX}}},B+t_{frac{alpha}{2}}frac{s}{sqrt{S_{XX}}}right]$ |
Correlation analysis
Correlation coefficient The correlation coefficient of two random variables $X$ and $Y$ is noted $rho$ and is defined as follows:
Sample correlation coefficient The correlation coefficient is in practice estimated by the sample correlation coefficient, often noted $r$ or $hat{rho}$, which is defined as:
Testing for correlation In order to perform a hypothesis test with $H_0$ being that there is no correlation between $X$ and $Y$, we use the following statistic:
Fisher transformation The Fisher transformation is often used to build confidence intervals for correlation. It is noted $V$ and defined as follows:

By noting $V_1=V-frac{z_{frac{alpha}{2}}}{sqrt{n-3}}$ and $V_2=V+frac{z_{frac{alpha}{2}}}{sqrt{n-3}}$, the table below sums up the key results surrounding the correlation coefficient estimate:
| Sample size | Standardized statistic | $1-alpha$ confidence interval for $rho$ |
| large | $displaystylefrac{V-frac{1}{2}lnleft(frac{1+rho}{1-rho}right)}{frac{1}{sqrt{n-3}}}underset{ngg1}{sim}mathcal{N}(0,1)$ | $displaystyleleft[frac{e^{2V_1}-1}{e^{2V_1}+1},frac{e^{2V_2}-1}{e^{2V_2}+1}right]$ |